Geometry
Reason With Shapes And Their Attributes.
3.G.A.1
Understand that shapes in different categories (e.g., rhombuses, rectangles, and others) may share attributes (e.g., having four sides), and that the shared attributes can define a larger category (e.g., quadrilaterals). Recognize rhombuses, rectangles, and squares as examples of quadrilaterals, and draw examples of quadrilaterals that do not belong to any of these subcategories.
Geometry
Draw And Identify Lines And Angles, And Classify Shapes By Properties Of Their Lines And Angles.
4.G.A.2
Classify two-dimensional figures based on the presence or absence of parallel or perpendicular lines, or the presence or absence of angles of a specified size. Recognize right triangles as a category, and identify right triangles.
Measurement And Data
Geometric Measurement: Understand Concepts Of Angle And Measure Angles.
4.MD.C.5
Recognize angles as geometric shapes that are formed wherever two rays share a common endpoint, and understand concepts of angle measurement:
Geometry
Classify Two-Dimensional Figures Into Categories Based On Their Properties.
5.G.B.3
Understand that attributes belonging to a category of two- dimensional figures also belong to all subcategories of that category. For example, all rectangles have four right angles and squares are rectangles, so all squares have four right angles.
Example Task
5.G Always, Sometimes, Never
Task
Decide whether each of these statements is always, sometimes, or never true. If it is sometimes true, draw and describe a figure for which the statement is true and another figure for which the statement is not true.
- A rhombus is a square
- A triangle is a parallelogram
- A square is a parallelogram
- A square is a rhombus
- A parallelogram is a rectangle
- A trapezoid is a quadrilateral
Solution
1. A rhombus is a square.
This is sometimes true. It is true when a rhombus has 4 right angles. It is not true when a rhombus does not have any right angles.
Here is an example when a rhombus is a square:

Here is an example when a rhombus is not a square:

2. A triangle is a parallelogram.
This is never true. A triangle is a three-sided figure. A parallelogram is a four-sided figure with two sets of parallel sides.
3. A square is a parallelogram.
This is always true. Squares are quadrilaterals with 4 congruent sides and 4 right angles, and they also have two sets of parallel sides. Parallelograms are quadrilaterals with two sets of parallel sides. Since squares must be quadrilaterals with two sets of parallel sides, then all squares are parallelograms.
4. A square is a rhombus
This is always true. Squares are quadrilaterals with 4 congruent sides. Since rhombuses are quadrilaterals with 4 congruent sides, squares are by definition also rhombuses.
5. A parallelogram is a rectangle.
This is sometimes true. It is true when the parallelogram has 4 right angles. It is not true when a parallelogram has no right angles.
Here is an example when a parallelogram is a rectangle:

Here is an example when a parallelogram is not a rectangle:

6. A trapezoid is a quadrilateral.
This is always true. Trapezoids must have 4 sides, so they must always be quadrilaterals.
Progressions
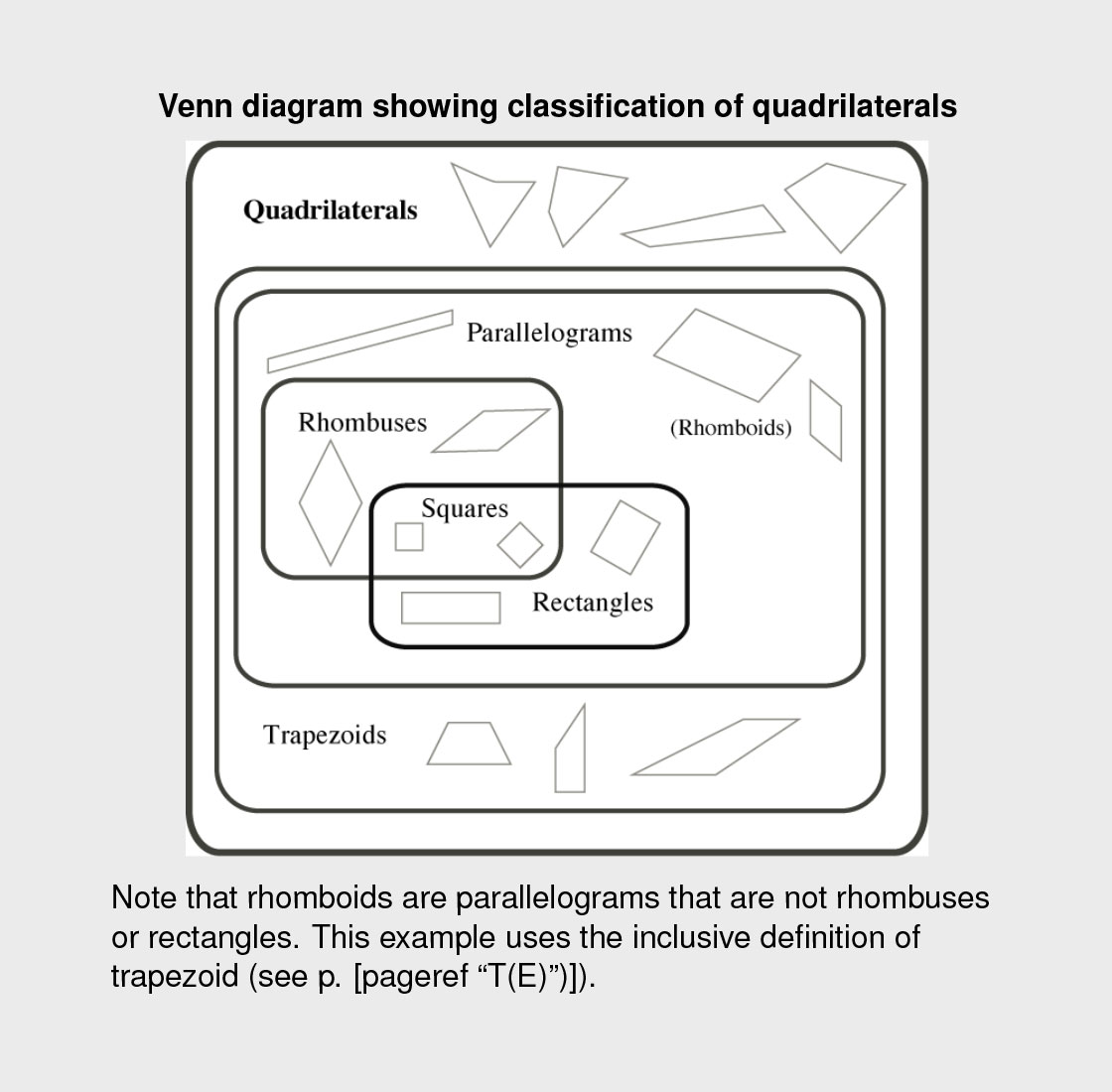
Note that rhomboids are parallelograms that are not rhombuses or rectangles. This example uses the inclusive definition of trapezoid.
Please reference page 18 in the Progression document.
Assessment Item
Focus
Geometry
Classify Two-Dimensional Figures Into Categories Based On Their Properties.
5.G.B.4
Classify two-dimensional figures in a hierarchy based on properties.