In my work as a Mathematics Specialist at Student Achievement Partners, I get the opportunity to talk to and work with educators from around the country. Often, especially in conversations with elementary school teachers, we talk about challenges with their instructional materials. Elementary teachers are responsible for so much content across multiple subjects, and so they really need instructional materials that set them and their students up for success. With this in mind, Student Achievement Partners has been working to create guidance documents for major publishers’ textbooks to show how to use them in ways that align to college- and career-ready standards. The purpose of the documents is to point out essential parts of the program and encourage teachers to de-emphasize content and activities that are less powerful for student learning or don’t align to the standards. Although we’ve now completed textbook-specific documents for GO! Math and enVisionmath 2.0, working with educators to create them has brought up some tweaks to materials that teachers can make with pretty much any math textbook they use.

Let the kids do the math!

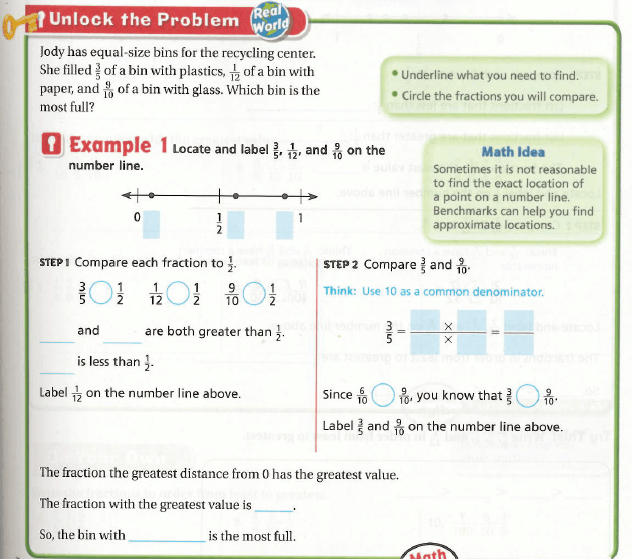
Across the board we see many textbooks that provide too much scaffolding for students as they complete math problems. Consider this problem at the beginning of a 4th grade lesson:

There are a lot of blanks, shaded squares, and circles that students are asked to fill in. The textbook developers were trying to lead students through a variety of strategies and models to help students compare the three fractions given. But at this point in 4th grade, students have had enough experience with fractions that they would likely come up with different ways of comparing fractions based on their reasoning and understanding of fractions. By giving just the problem (without all of the hints and suggested methods), students have the opportunity to make sense of the mathematics and the teacher has the opportunity to use different strategies students generate to facilitate a meaningful class discussion that’s based on mathematical reasoning.

Quick Fix: Project just the problem and have students solve it in a notebook, rather than using the printed textbook pages.

Teach the math, not the model or the strategy

Good mathematicians need to be able to use models and strategies based on the problem in front of them.  Unfortunately, some textbooks have interpreted this to mean that students need to learn all of the possible models or strategies. Teachers and students can get lost in naming strategies and models, or being asked to show different strategies just for the sake of variety.

This grade 1 table of contents shows how this issue plays out in textbooks.

The grade 1 standard, 1.NBT.C.4 says: Add within 100, including adding a two-digit number and a one-digit number, and adding a two-digit number and a multiple of 10, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used. Understand that in adding two-digit numbers, one adds tens and tens, ones and ones; and sometimes it is necessary to compose a ten. The last sentence highlights the conceptual understanding 1st graders need in order to generalize and add larger numbers over the next couple of years. However, this list of lessons looks like a checklist of possible models and strategies without an acknowledgment of the mathematical concept underlying this standard.

Quick Fix:   Remove the expectation to use a specific strategy or model from tasks and problems. Take a look at this teacher’s quick adaptation of a topic assessment question:

Doing this is simple, quick, and allows the teacher to see how students are approaching adding 2-digit numbers.

Pay attention to aspects of Rigor

We know that the elements of Rigor (conceptual understanding, procedural skill and fluency, and application) are all equally valuable to students’ mathematical power. (See the Final Report of the National Mathematics Advisory Panel from 2008 for an end to the ‘math wars’ that represented the debate over which aspect was most important.) Sometimes this understanding (that all aspects are equally important) results in including all three aspects of Rigor in each lesson. However, that’s not what the Standards require. One surprising example of this: the 3rd grade Numbers and Operations – Fractions standards do not call for application at all. (Go ahead and re-read them!)  So while pizzas and pans of brownies allow students to connect the partitioning work of grade 1 and 2 to the grade 3 fraction work, they don’t help so much with the cluster heading for 3.NF: Develop understanding of fractions as numbers. It’s worth taking a close look at how your textbook treats this cluster. Are all fraction problems in context? Do students have enough work with fractions on a number line? There might be some adjustments needed to ensure the aspect of Rigor being targeted aligns with the standards. Teachers can make choices about which problems to spend both instructional and assessment time on based on the language of the standards.

The mismatch on Rigor often comes up in chapter tests.  This end of chapter assessment comes from a grade 5 chapter called “Fluently Multiply Multi-Digit Numbers:”

However, no problem on this page of this assessment actually assesses the fluency required by 5.NBT.B.5 Fluently multiply multi-digit whole numbers using the standard algorithm.

Quick Fix: Start with the end in mind! Review the chapter test and if they contain too many problems that stray from the aspect of Rigor called for by the standards: Delete, delete, delete. Use this to inform choices you make within individual lessons.

The work of an elementary teacher seems endless and creating materials shouldn’t be on that list. Rather than turning to Google, give these Quick Fixes a try to improve your students’ mathematical experiences. With some combination of the them, most textbooks can be adapted to better support students in reaching the mathematical promise of the standards.