Editor’s Note: Jason Zimba, one of the lead writers of the Common Core State Standards for Mathematics, has instituted something he calls Saturday School for his daughters. Each Saturday, Zimba and his wife engage their two daughters with fun activities devised to not only bolster the girls’ math skills, but also instill a true enjoyment of math. In a recent post on his personal blog, Zimba describes how he uses flashcards. Whether done at home or in the classroom, when used properly, flashcards can be a fun and effective way for students to build fluency and fact recall.
This article originally appeared under the title “How We’ve Been Using Flashcards” on Jason Zimba’s blog here.
Saturday School is never very long, but over time we still manage to do a range of things, including solving word problems, learning concepts, and doing exercises that build fluency and fact recall. Our materials include worksheets that I create, workbooks off the shelf such as Kyoiku Dojinsha and others, released test questions, homemade flashcards, and pennies or dice that we might use to play a math game.
Concerning flashcards in particular, a researcher in mathematics education saw this article of mine and later emailed me an important tip that I wanted to pass along:
[Flashcards] … are a good fluency method [but] please stress that students should be spending most of their time on the cards they do not know yet or on those they know but are not fast on yet. Most drill uses many problems students know and thus is a big time waster.
A similar message appears in this document for educators:
Organizing practice so that it focuses most heavily on understood but not yet fluent products and unknown factors can speed learning. To achieve this by the end of Grade 3, students must begin working toward fluency for the easy numbers as early as possible.
Time is always scarce, and it is valuable to customize flashcard work to the student’s current state of mastery. Some digital apps do allow you to adjust the settings so that students are focusing on the facts they need to focus on—maybe some of them even adjust themselves automatically over time. In any case, here’s how I’ve tried to accomplish something similar using old-fashioned flashcards. I make no claim that my method is the best, or even better than others! But it has worked for us, and it’s been fun.
Here’s how it works:
- The flashcards are kept in a “piggy-bank” made from an old tissue box.
- We work at the dining table. My wife or I will draw a flashcard from the bank and show it.
- If the answer comes back “lickety-split,” then the card is set aside. Otherwise, the card goes back in the bank. If there is doubt, then the card goes back in the bank.
- If the student is drawing a complete blank, then I prompt with a strategy, for example if the problem is 6×8 and the reaction is a blank stare, then I might prompt with “Do you know 5 × 8?” Then the student can say “Oh right, that’s 40, so 8 more is 48.” Of course, the card goes back in the bank, but it has been a good just-in-time learning opportunity.
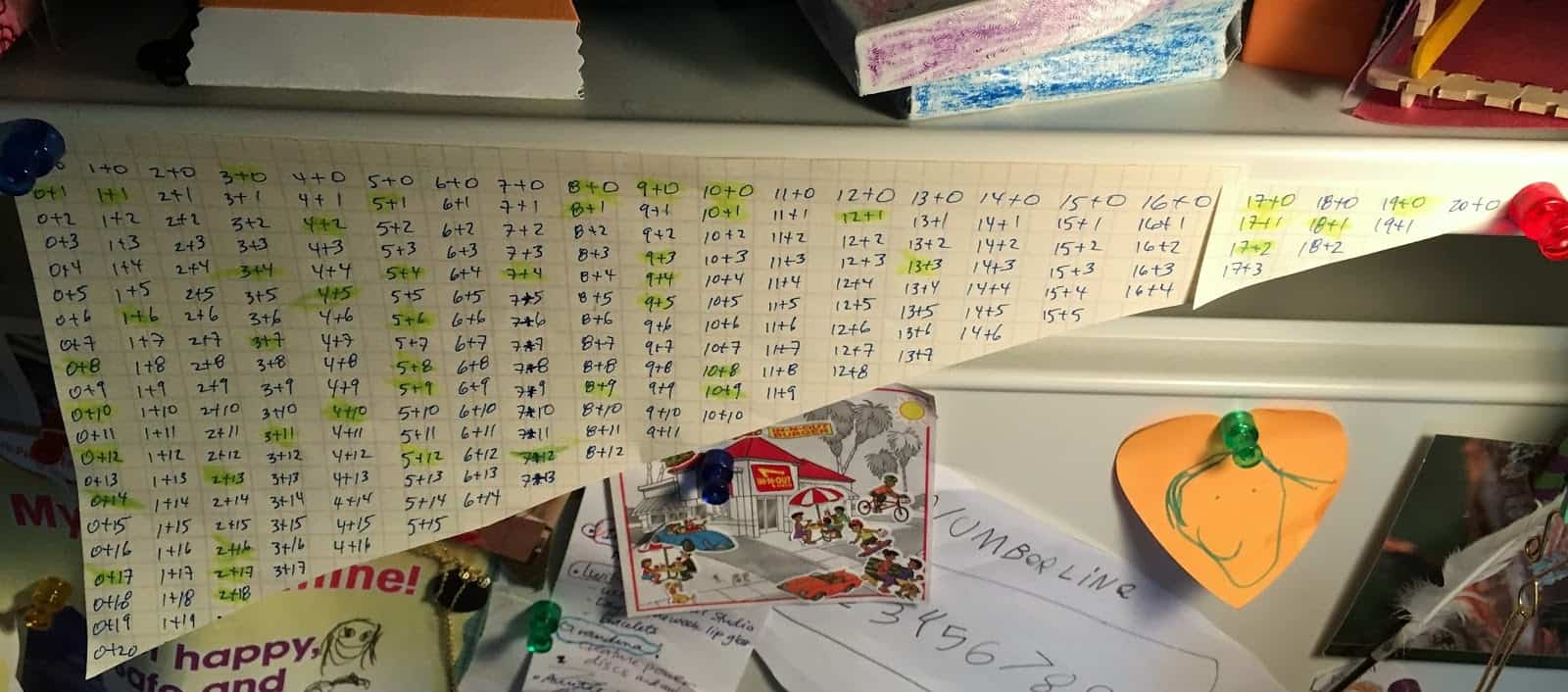
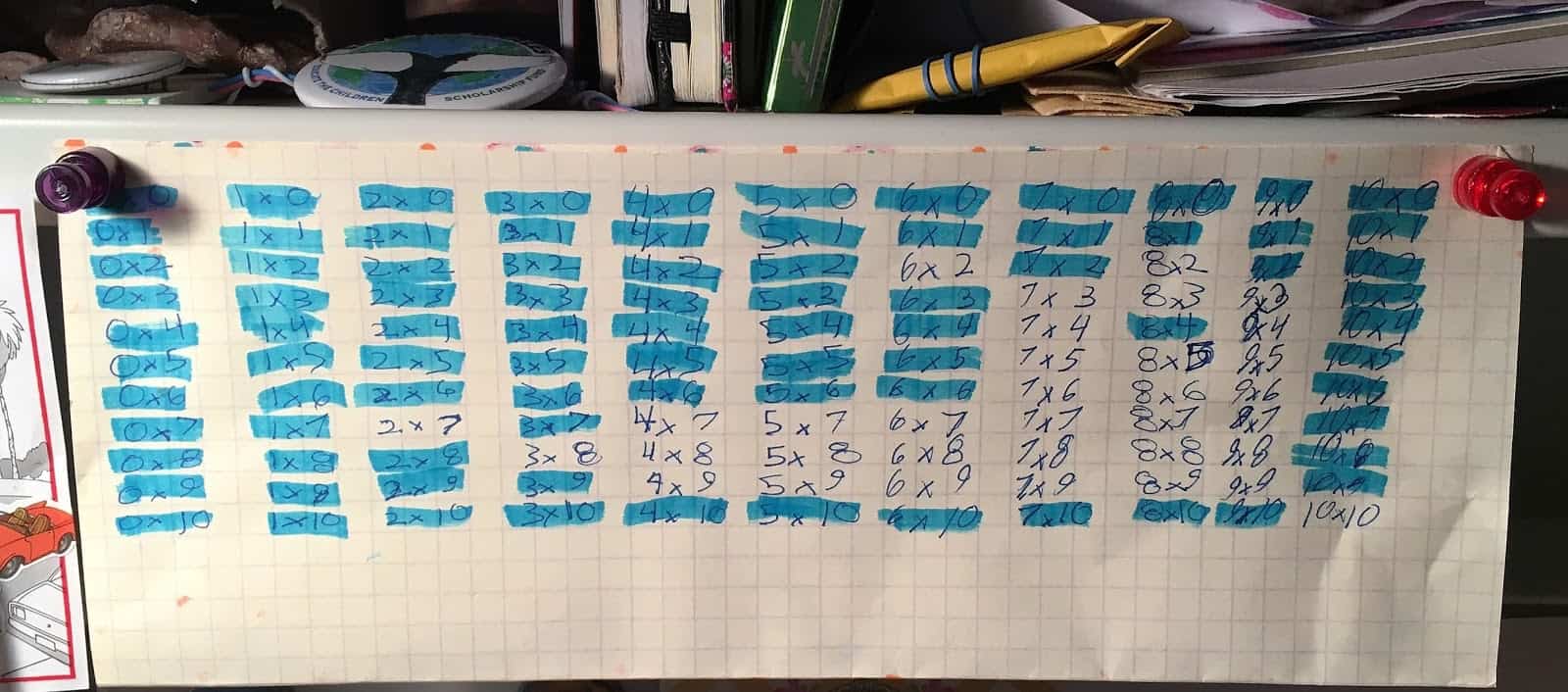
We’ll do anywhere from 5 to 15 minutes of this, and at the end, the student “owns” all of the cards that have been set aside. The student highlights the known facts on a map (images below) and puts the cards into a keepsake box.
This work proceeds in tandem with our other activities, including worksheet practice on facts (customized according to what the map says). Eventually, the day comes when there are no more cards left in the bank and the entire map has been highlighted. Time to celebrate! From then on we’ll still do occasional maintenance practice to keep the facts secure (worksheets or a configurable app), and of course there are the worksheets that come home from school.
The way the system works is that the bank gets emptier and emptier over time, with known facts exiting the process as they become known. That’s how the game exemplifies the advice I shared at the outset about customizing fact practice.
Although initially the bank contains many already-known facts like 2 + 1 or 3 + 0, this is intentional in order that the early sessions will feature easy, known facts and establish a foundation of confidence as we embark on the process. The rapid progress on Day 1 creates excitement. Pretty soon, those easy known facts exit the system and the bank becomes nicely focused on the student’s individual horizon. Of course, this also means that the work is getting tougher over time, so I pay attention to motivation and emotions while we work.
(The addition map is like the one I showed in this post.) We use magnets to pin the maps to metal shelves above the kids’ desks. Each weekend, they chart new progress using a highlighter.The maps are a great way to recognize accomplishment. The kids love adding to the maps and seeing their maps fill up over time. Completing the map brings a strong sense of satisfaction and achievement.For my purposes, the map also suggests hypotheses about where prerequisite concepts might be lacking. I can address those directly in a separate line of work.Here’s what our flashcards look like. They are made out of ordinary 3×5 note cards. (To save paper, I write several problems on each note card and then cut them out with scissors.)
Now, the setup is actually a little different depending on whether we’re talking about addition or multiplication. Let’s consider addition first. By the time we’re doing this, the student can mentally calculate all or almost all of the sums on the map, but some of the facts are very slow, and not too many of the facts are known from memory. So after I have written out all of the sums, I give the flashcards to the student and she goes through them one by one, writing each answer on the back of the card.With the cards complete, we’re ready for the funnest part: turning an empty tissue box into a bank. Here is a picture of last year’s “piggy bank” of addition facts (piggy-posterior not shown).
Multiplication differs from addition in the setup, because at the time when we first create the flashcards, the student doesn’t really know how to calculate all of the products yet. Products only go into the bank if the student has seen them before and can calculate them mentally (perhaps slowly in some cases). I did a little probing at the outset to find out roughly where we were.
So that’s how we’ve used flashcards in Saturday School. Readers might wonder why we only included sums and products, since students also have to be fluent with differences and quotients. Including differences and quotients is good, and I also like flashcards that show the entire fact family (you cover up two of the numbers, and the student tells you the third). In my case however, it happens that the kids are so secure with the relationships between operations that, presented with 14 − 9 or 24 ÷ 6, they just answer by consulting their mental “lookup table” of + or ×. They also get direct practice with differences and quotients in worksheets that I give them or that come home from school.



















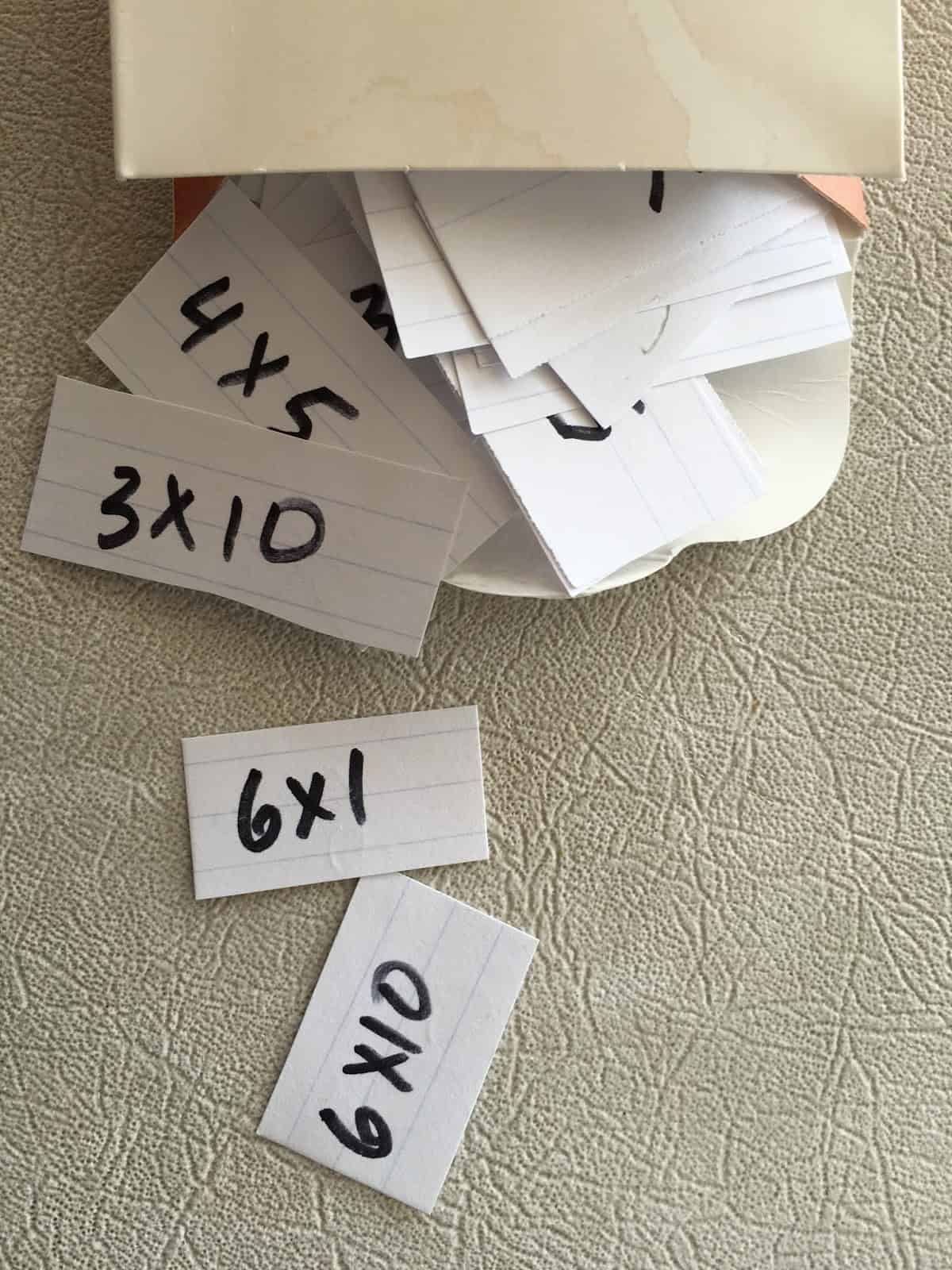

Please stop encouraging the use of flashcards! It does not improve fluency (at least not in any meaningful way). It only encourages students to memorize answers. Even worse, it teaches them that being good at math means being able to recall facts quickly. That is not what math should be about and it is not the way people who are truly skilled at math do it. Math is about logic and patterns. As teachers, we need to emphasize critical thinking skills rather than memory techniques. Who cares if it takes a child a few seconds to figure out what 8 times 7 is? It is much better to give them a solid foundation of number sense before they attempt more advanced math. As they progress, they will become faster with trivial facts. If they learn to memorize the trivial facts they will attempt to use the same technique to learn algebra and geometry and it will become more and more difficult to keep up. The alternative is to build on their logic skills so that learning more advanced math becomes easier, not harder. I have had students who struggle to tell me what half of two is because they are trying to remember the answer. I refused to memorize the times tables when I was seven years old because I realized I could just figure it out. I didn’t win any of the silly flashcard games we played in elementary school but years later I earned a PhD in physics. I would not have been able to accomplish that if I had tried to learn math by memorizing facts. Again, I beg you, please stop encouraging people to use flashcards! They are the worst thing that has ever happened to math education. If you want a better way to teach math, check out the work of Dan Meyer.
“To prepare students for Algebra, the curriculum must simultaneously develop conceptual understanding, computational fluency, and problem-solving skills. Debates regarding the relative importance of these aspects of mathematical knowledge are misguided. These capabilities are mutually supportive, each facilitating learning of the others. Teachers should emphasize these interrelations; taken together, conceptual understanding of mathematical operations, fluent execution of procedures, and fast access to number combinations jointly support effective and efficient problem solving.”
-National Mathematics Advisory Panel, 2008
Great article thank you for it. A question regarding flashcard format. Do you recommend the horizontal format to the vertical? Or does it really matter?
I always say horizontal…students need to see the problems written that way to discourage them from always wanting to “line up” the problems. This helps the students think about the numbers first before jumping to any particular strategy. Just my $0.02.
Thx
A huge factor overlooked by those that believe memorization inhibits fluency is learning style ie: visual vs verbal learners. Verbal learners need to memorize the answers. Showing the answer in pictures to a verbal learner is not the same as showing it in pictures to a visual learner.