Research and Reflections

What Really Counts When We Teach?

What should we look for in powerful classrooms?

Alan Schoenfeld
By: Alan Schoenfeld
Posted:

There’s so much to do. What should we focus on?

Lots of things matter. You need a rich curriculum. You’ll be driven crazy if the assessments aren’t aligned, and things will be difficult if administrators don’t support what you’re doing. But let’s narrow down to your teaching. Say you had to pick five things to focus on when planning to teach. What would they be?

The Teaching for Robust Understanding (TRU) framework (see Figure 1) highlights five essential dimensions of teaching. If you get these right, your students will be knowledgeable and resourceful thinkers and problem solvers.

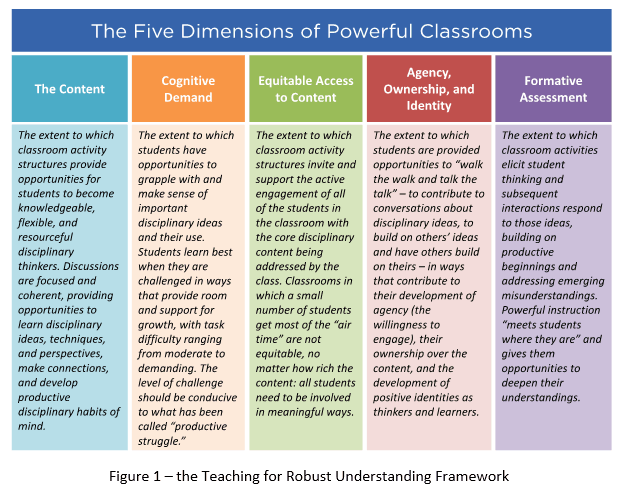Dimensions 2 through 5 focus on the student experience. That shouldn’t come as a surprise: it’s the students who are doing the learning, after all. So, they’d better have opportunities for sense making, at a level that they can engage with. If things are too easy, or too hard, they can’t engage in “productive struggle” (dimension 2, cognitive demand). We want all students to have meaningful opportunities to learn (dimension 3 equitable access to content) – and, opportunities to do so in which they get to refine their ideas in collaboration with others, in ways that allow them to see themselves as disciplinary thinkers (dimension 4, agency, ownership, and identity). After all, a positive disciplinary identity, whether as a writer, a scientist, a historian – you name it! – comes from engaging meaningfully with the discipline. And, of course, if the students reveal what they know, it’s much easier to meet them where they are. That’s what formative assessment (dimension 5) is all about. There’s loads of documentation for these claims; see [References 1,2]. More important, there are tools to help get better at this – see an introduction at [Reference 3] and tools such as these conversation guides [References 4,5] and observation guides [References 6,7].

How much do disciplinary knowledge and disciplinary tools (for example, observation rubrics) matter? After all, don’t four of the five TRU dimensions look discipline-free?

Well, they may look that way, but they’re not. That’s because each of the dimensions lives by way of the disciplinary opportunities made available to the students—and how that happens in math, for example, is very specific to mathematics.

I’ll illustrate this using my own disciplinary strengths and weaknesses. I’m pretty good at math, and I have a lot of what’s been called “pedagogical content knowledge” in math – I know what the implications of student thinking are; I know what ideas are productive, and which ones lead to dead ends; I have a good understanding of what it means to connect mathematical ideas. I “get” the standards, so I can help make them come alive in my classroom.  I might not do too badly at ELA because I’ve spent a lot of time working on related issues. But, I don’t have a deep enough understanding of social studies or history to help my students experience those disciplines in rich ways, and when it comes to some of the sciences, well, forget it – my knowledge is superficial. I could master the facts and formulas, but when it comes to supporting deep understanding in my students… you’d want someone who really gets it, not me.

What does this say about TRU, and about observation tools in general? Knowledge of the discipline, and of student thinking in it, become central as soon as you dig into each dimension. How do I know what’s easy or hard (cognitive demand)? How do I know where students can gain entry into the content (equitable access), or debate productively (agency, ownership, identity)? How do I know what to listen for, and how to adjust my instruction? The more I know about the content and student thinking in it, the better I can address these issues.

In fact, deep disciplinary understanding plays out with regard to curriculum, assessment, and coaching and classroom observations. The best curriculum materials I know pay deep attention to the content riches, and to student thinking. Take a look at the Formative Assessment Lessons at the Mathematics Assessment Project website [Reference 8]. What makes them so powerful [References 9,10] is that they’re aimed at deep understanding of the content, and grounded in an understanding of the things students are likely to understand and misunderstand. Regarding assessment, Hugh Burkhardt’s phrase WYTIWYG – “What You Test Is What You Get – is all too true. Dumbed-down tests aim us in the wrong directions, while rich tests help us measure what matters. And if I’m to have help from colleagues when I want to improve my teaching, what I’d like are colleagues who “get” both the content I’m teaching and student thinking in the discipline. I need tools that help in similar ways – tools that help me focus on what counts in my classroom. Compelling arguments in mathematics (“proofs”) are different from rigorous arguments in literature, or in science classrooms, for example. Misconceptions are content-specific. Tools are more powerful if they’re tailored to my needs. So, let’s focus on the big ideas, such as the TRU dimensions – AND, let’s build more discipline-specific tools, such as observation tools and discussion guides, that help us dig into the specifics of what we teach.

References

[1]  Schoenfeld, A. H. (2013). Classroom observations in theory and practice. ZDM, the International Journal of Mathematics Education, 45: 607-621. DOI 10.1007/s11858-012-0483-1.

[2] Schoenfeld, A. H. (2014, November). What makes for powerful classrooms, and how can we support teachers in creating them? Educational Researcher, 43(8), 404-412. DOI: 10.3102/0013189X1455

[3]  Schoenfeld, A. H., & the Teaching for Robust Understanding Project. (2016). An Introduction to the Teaching for Robust Understanding (TRU) Framework. Berkeley, CA: Graduate School of Education. Retrieved from http://map.mathshell.org/trumath.php or http://tru.berkeley.edu.

[4]  Baldinger, E. Louie, N., and the Algebra Teaching Study and Mathematics Assessment Project. (2016). TRU Math conversation guide: A tool for teacher learning and growth (mathematics version). Berkeley, CA & E. Lansing, MI: Graduate School of Education, University of California, Berkeley & College of Education, Michigan State University. Retrieved from:  http://ats.berkeley.edu/tools.html and/or http://map.mathshell.org/materials/pd.php.

[5] Louie, N., Baldinger, E. and the Algebra Teaching Study and Mathematics Assessment Project. (2016). TRU Math conversation guide: A tool for teacher learning and growth (Domain-general version). Berkeley, CA & E. Lansing, MI: Graduate School of Education, University of California, Berkeley & College of Education, Michigan State University. Retrieved from:  http://ats.berkeley.edu/tools.html and/or http://map.mathshell.org/materials/pd.php.

[6]  Schoenfeld, A. H., and the Teaching for Robust Understanding Project. (2016b). The Teaching for Robust Understanding (TRU) observation guide for mathematics: A tool for teachers, coaches, administrators, and professional learning communities. Berkeley, CA: Graduate School of Education, University of California, Berkeley. Retrieved from:  <http://TRU.berkeley.edu> or <http://map.mathshell.org/> or <http://ats.berkeley.edu/>.

[7]  Schoenfeld, A. H., and the Teaching for Robust Understanding Project. (2016a). The Teaching for Robust Understanding (TRU) observation guide: A tool for teachers, coaches, administrators, and professional learning communities. Berkeley, CA: Graduate School of Education, University of California, Berkeley. Retrieved from:  <http://TRU.berkeley.edu> or <http://map.mathshell.org/> or <http://ats.berkeley.edu/>.

[8]  Mathematics Assessment Project. Formative Assessment Lessons, at http://map.mathshell.org/lessons.php.

[9]  Herman, J., Epstein, S., Leon, S., La Torre Matrundola, D., Reber, S., & Choi, K. (2014). Implementation and effects of LDC and MDC in Kentucky districts (CRESST Policy Brief No. 13). Los Angeles: University of California, National Center for Research on Evaluation, Standards, and Student Testing (CRESST).

[10] Research for Action. (2015).  MDC’s Influence on Teaching and Learning. Philadelphia, PA: Author.  Retrieved March 1, 2015 from https://www.researchforaction.org/publications/mdcs-influence-on-teaching-and-learning/

Save

Tags:

Leave a Reply

Your email address will not be published.

About the Author: Alan Schoenfeld is the Elizabeth and Edward Conner Professor of Education and Affiliated Professor of Mathematics at the University of California at Berkeley. He is a Fellow of the American Association for the Advancement of Science and of the American Educational Research Association (AERA), and a Laureate of the education honor society Kappa Delta Pi; he has served as President of AERA and vice President of the National Academy of Education. He holds the International Commission on Mathematics Instruction’s Klein Medal, the highest international distinction in mathematics education; AERA's Distinguished Contributions to Research in Education award, AERA’s highest honor; and the Mathematical Association of America’s Mary P. Dolciani award, given to a pure or applied mathematician for distinguished contributions to the mathematical education of K-16 students. Schoenfeld’s main focus is on Teaching for Robust Understanding. A Brief overview of the TRU framework, which applies to all learning environments, can be found at The Teaching for Robust Understanding (TRU) Framework. A discussion of TRU as it applies to classrooms can be found in What makes for Powerful Classrooms?, and a discussion of how the framework can be used systemically can be found in Thoughts on Scale. Schoenfeld's research deals broadly with thinking, teaching, and learning. His book, Mathematical Problem Solving, characterizes what it means to think mathematically and describes a research-based undergraduate course in mathematical problem solving. Schoenfeld led the Balanced Assessment project and was one of the leaders of the NSF-sponsored center for Diversity in Mathematics Education (DiME). The DiME Center was awarded the AERA Division G Henry T. Trueba Award for Research Leading to the Transformation of the Social Contexts of Education. He was lead author for grades 9-12 of the National Council of Teachers of Mathematics' Principles and Standards for School Mathematics. He was one of the founding editors of Research in Collegiate Mathematics Education, and has served as associate editor of Cognition and Instruction. He has served as senior advisor to the Educational Human Resources Directorate of the National Science Foundation, and senior content advisor to the U.S. Department of Education's What Works Clearinghouse. He was also one of the lead authors for the mathematics content specifications for the Smarter Balanced Assessment Consortium. In his essay, Common Sense About the Common Core, Schoenfeld answers key questions and clears up common misconceptions about Common Core's mathematics standards. Schoenfeld has written, edited, or co-edited twenty-two books and approximately two hundred articles on thinking and learning. He has an ongoing interest in the development of productive mechanisms for systemic change and for deepening the connections between educational research and practice. His most recent book, How we Think, provides detailed models of human decision making in complex situations such as teaching, and his current research focuses on the attributes of classrooms that produce students who are powerful thinkers. Schoenfeld's current projects (the Algebra Teaching Study, funded by NSF; the Mathematics Assessment Project (MAP) and Formative assessment with Computational Technologies (FACT), funded by the Gates Foundation; and work with the San Francisco and Oakland Unified School Districts under the auspices of the National Research Council's SERP project) all focus on understanding and enhancing mathematics teaching and learning.