• Making the Shifts

  How does this task exemplify the instructional Shifts required by CCSSM?

  Focus	Belongs to the major work of fifth grade
  Coherence	Builds on key understandings of multiplying a fraction by a whole number (4.NF.B.4); lays foundation for division of fractions by fractions (6.NS.A)
  Rigor	Conceptual Understanding: primary in this task Procedural Skill and Fluency: not targeted in this task Application: primary in this task

• Task

  

  Carolina is making her special banana pudding recipe. She is looking for her cup measure, but can only find her quarter cup measure.

  1. How many quarter cups does she need for the sour cream? Draw a picture to illustrate your solution, and write an equation that represents the situation.
  2. How many quarter cups does she need for the milk? Draw a picture to illustrate your solution, and write an equation that represents the situation.
  3. Carolina does not remember in what order she added the ingredients but the last ingredient added required 12 quarter cups. What was the last ingredient Carolina added to the pudding? Draw a picture to illustrate your solution, and write an equation that represents the situation.
• Illustrative Mathematics Commentary and Solution

  Commentary:

  The purpose of this task is to provide students with a concrete situation they can model by dividing a whole number by a unit fraction. For students who are just beginning to think about the meaning of division by a unit fraction (or students who have never cooked), the teacher can bring in a 1/4 cup measuring cup so that students can act it out. If students can reason through parts (a) and (b) successfully, they will be well-situated to think about part (c) which could yield different solution methods.

  Students may need a great deal of practice seeing the connection between the visual representation and the more abstract equations. This task provides an excellent opportunity for teachers to emphasize the relationship between multiplication and division, and if students do not automatically see the connection, the teacher should draw their attention to it.

  The approaches described in the solution can be used to make the connection between dividing by a whole number and multiplying by its reciprocal. If students are just beginning to understand the meaning of dividing by a unit fraction, such a conversation should be postponed. However, if they feel comfortable solving these kinds of problems with pictures, this task provides a perfect opportunity to see that dividing a whole number by a unit fraction is the same as multiplying by the reciprocal of the unit fraction. This, in turn, prepares them for the more general result that dividing by any fraction is the same as multiplying by its reciprocal, which students will see in 6th grade.

  Solution:

       a. Carolina needs 8 quarter cups of sour cream because there are 4 quarter cups in 1 cup, and so it takes $2×4=8$ quarter cups to make 2 cups:

  
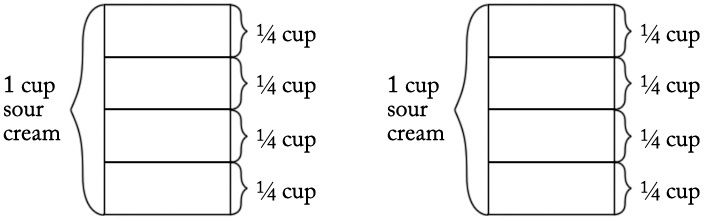
  This is a "how many groups?" division problem because it asks "How many quarter cups are in 2 cups?"
  There are two correct equations:

  $2÷\frac{1}{4}=?$

  
  or equivalently:

  $?×\frac{1}{4}=2$

  
  We can verify that 8 is the correct solution by noting that $8×\frac{1}{4}=\frac{8}{4}=2$ (4.NF.B.4c)

       b. Carolina needs 16 quarter cups of milk because there are 4 quareter cups in 1 cup and so $4×4$ quarter cups in 4 cups, as we see in this picture:
  
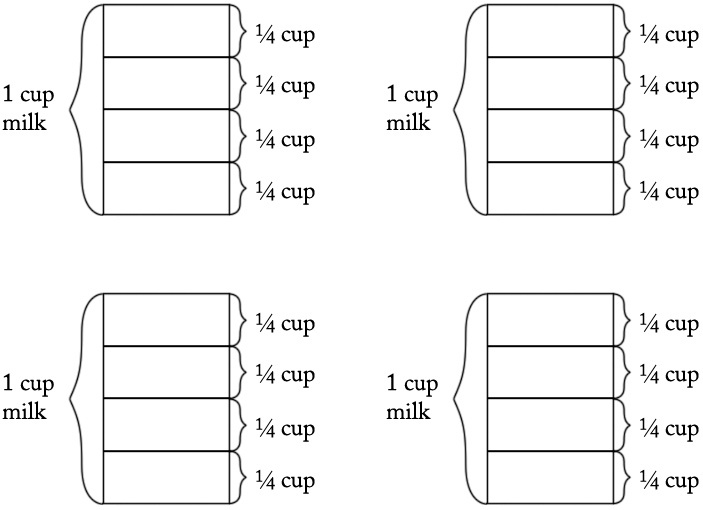
  Again, this is a "how many groups?" division problem because it asks "How many quarter cups are in 4 cups?" There are two correct equations:

  $4÷\frac{1}{4}=?$

  
  or equivalently:

  $?×\frac{1}{4}=4$

  
  We can verify that 16 is the correct solution by noting that $16×\frac{1}{4}=\frac{16}{4}=4$

       c. We can think of this problem several ways. First, we can ask, "How many cups did I start with if i ended up with 12 quarter cups?" We could write an equation for this:

  $?÷\frac{1}{4}=12$

  
  We could also say, "I have 12 quarter cups and I want to know how many cups this is, so I can multiply 12 and $\frac{1}{4}$ to find the number of cups I started with." This can be represented with the following equation:

  $12×\frac{1}{4}=?$

  
  Notice that these two equations are equivalent and can both be interpreted in terms of the following picture: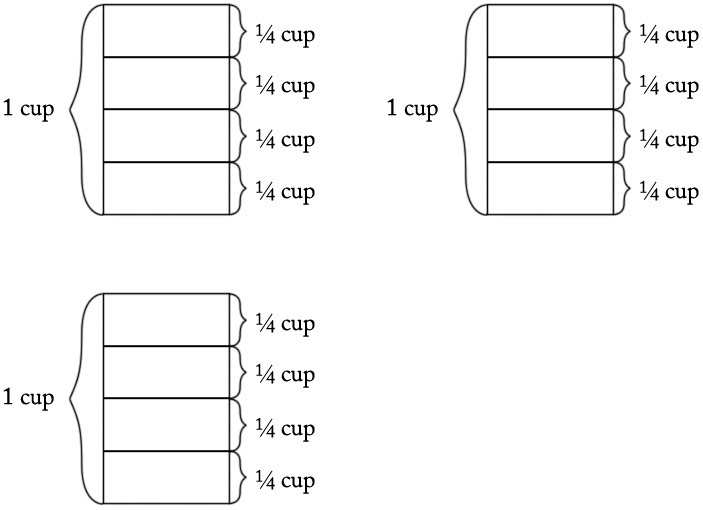
  
  Here is yet another approach: If we note that there are 4 quarter cups in 1 cup, we can also think of this as a "how many groups?" division problem. We know that there are a total of 12 quarter cups and that there are 4 quarter cups in a cup (a group), and we want to know how many cups (or how many groups) this makes. Here the equation is:

  $12÷4=?$

  
  Since $12÷4=3$, she must have started with the vanilla pudding mix, as that is the only ingredient that requires 3 cups.

• Additional Thoughts

  The relationship between multiplication and division (3.OA.B.6) and understanding fractions as numbers (3.NF.A) are both developed in third grade and extended in fourth grade. The problems and the equations students are asked to generate in this task provide an opportunity for students to extend their understanding of the operations with whole numbers they learned in third grade to multiplying a whole number by a fraction.

  Students may use a variety of solution methods to solve the questions in this task. No matter what methods students use to solve the problems in this task, they are asked to write equations to describe the mathematics. This allows all students, even those who find opportunistic methods to solve problems, a chance to connect their thinking to the mathematics they are using.  Comparing students’ equations – those that used multiplication with those that used division - provides the class with a preview of dividing fractions which they will begin in grade 6.

  This task can also be used to introduce students to division of whole numbers by unit fractions.   It is likely that students will use prior knowledge of multiplication of whole numbers and fractions (4.NF.B.4) to solve these problems. When students use multiplication to solve these problems, it provides an opportunity for the teacher to illustrate the relationship between multiplication and division using a visual model such as a number line to show how two wholes divided into fourths equals eight fourths total. Thus, students are able to see how $\frac{1}{4}×8=2$ is related to $2÷\frac{1}{4}=8$.

  The concepts, skills, and problem solving involved in multiplying and dividing fractions is major work for grade 5. For more insight on performing these operations with fractions, read pages 12–14 of the progression document, 3–5 Number and Operations – Fractions.

Send Feedback