In this six-part blog series, Chrissy Allison and Becca Varon will illustrate how to make some of the trickiest standards in grades 6-8 accessible for all students and provide concrete examples of how math educators can address unfinished learning within the context of grade-level lessons, which in the long term will help prevent an entrenched pattern of over-remediation and below-grade-level teaching. You can read their introductory post here.

In this second post, we will explore ways to “bridge the gap” with the 6th-grade standard 6.NS.A.1. Let’s take a look at the standard itself. As you read, consider how it compares to the teaching methods and strategies you use:

Apply and extend previous understandings of multiplication and division to divide fractions by fractions. 6.NS.A.1: Interpret and compute quotients of fractions, and solve word problems involving division of fractions by fractions, e.g., by using visual fraction models and equations to represent the problem. For example, create a story context for (2/3) ÷ (3/4) and use a visual fraction model to show the quotient; use the relationship between multiplication and division to explain that (2/3) ÷ (3/4) = 8/9 because 3/4 of 8/9 is 2/3. (In general, (a/b) ÷ (c/d) = ad/bc.) How much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 3/4-cup servings are in 2/3 of a cup of yogurt? How wide is a rectangular strip of land with length 3/4 mi and area 1/2 square mi?.

We’ll never forget attending a math PD years ago where the facilitator told us that the soon-to-be-released Common Core State Standards were different: they demanded understanding, not just answer-getting. She then asked the room full of teachers to invent a real-world scenario that called for dividing a fraction by a fraction. The group really struggled to come up with anything despite the fact that many of us taught fraction division!

This 6th-grade standard, which is in the Major Work of the Grade, always reminds us of how far we’ve come since then—it’s rich with examples, and it requires flexible thinking and authentic application. In the past, we might have used tricks like “keep, change, change” to flip a division problem into a multiplication one. That’s not enough to meet this standard. Let’s dissect it to understand it more deeply:

Now, let’s look at an example task from Illustrative Mathematics that aims to build from conceptual understanding towards procedural skill. I found solving this task illuminating, and I encourage you to pick up a pencil and do the math:

I love how the progression of questions in this task illustrates the intention of the cluster to “apply and extend” prior understanding. Part a gets students thinking about division with whole numbers, which is work they started in third grade. Fifth-graders also learn how to divide unit (numerator of 1) fractions by whole numbers, and vice versa, which comes up in parts b and c.

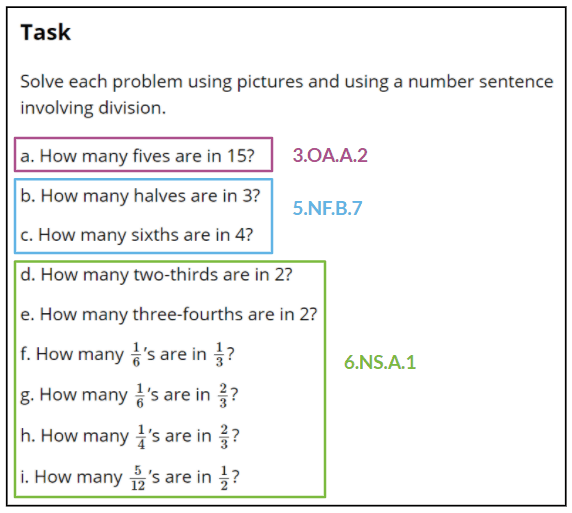
This task is beautifully designed to help students wake up their prior knowledge about division. Its structure provides a nice on-ramp to dividing fractions, and the first few questions might be enough to clear up lingering misconceptions and ensure everyone remembers how to represent division using pictorial models.

But what if we’ve diagnosed that the on-ramp will feel really steep for a large percentage of our students? By this, I mean that it might be their first experience with fraction division, or division of any kind. For this standard, we might think of this unfinished learning as being like a raised drawbridge that we need to close in order to proceed. In the past, when I’ve neglected to build this conceptual understanding, I’ve noticed that students are more likely to wind up memorizing a procedure that feels like a magic trick to them and doesn’t stick long-term. Investing extra time to “close the bridge” can be worth it, and it sometimes takes no more than 20-30 minutes.

There are a number of ways you might take action to address this unfinished learning during the first few parts of this task:

1. Make it concrete: Break out the manipulatives (even something as simple as cups of dried beans) for students to experiment with whole-number division. Discuss how quotients can answer questions that are partitive (how many in each group?) and quotitive (how many groups?). Then, you might use paper or virtual fraction strips, Play-Doh “snakes,” or even pieces of chewing gum to experiment with dividing whole numbers by unit fractions. You might also choose to ground discussion in a real-world scenario that students will find intriguing (example: this 3-Act Task.)
2. Have students compare and connect their representations: In each part of the task, pause to elevate a few student drawings. Ask students to discuss the similarities and differences they see. For example, you might ask, “Where can you see the dividend in each of these pictures?” Then, give them an opportunity to try out some of their peers’ ideas.
3. Provide students with more practice opportunities: Come prepared with a plan for where you might pause and invest more time if students continue to struggle. For example, if I were teaching this standard to a group of students with shaky understanding of whole-number division, I might invest up to a full day solidifying it using the ideas above. (However, it’s also important to get students access to grade-level work as quickly as possible; this is one of a truly limited number of topics where this type of time investment might be necessary.)

We hope this example was helpful as you work to “bridge the gap” to grade-level math. In the comments, please share how your students responded to the adapted task, additional scaffolding ideas, and any feedback you have. 

For more examples, be sure to check out other forthcoming posts on 7th- and 8th-grade standards in the Unfinished Learning in Middle School Mathematics series.